Factors affecting period of satellite.
Time period of a satellite formula.
Time period of satellite.
If you know the satellite s speed and the radius at which it orbits you can figure out its period.
Time period of a satellite.
T 2π r g 5 08 10 3 s 84 min.
Here r r h.
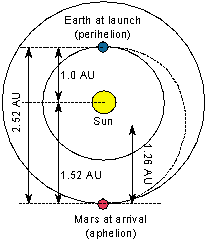
As long as the satellite maintains a circum solar orbit 10 2020 06 03 03 55 male 60 years old level or over an engineer.
T 2πr v 0 2π r h v 0.
Where p is the average density of earth.
Orbital velocity expression 2 step by step derivation for a mass of m on earth s surface the following is true.
We ll also solve sample numerical problem here using this law.
Artificial satellites are of two types.
The period of a satellite is the time it takes it to make one full orbit around an object.
The equation does not contain the term m which shows that the critical velocity is independent of the mass of the satellite.
The square of the time period of the satellite is directly proportional to the cube of the radius of orbit r of the satellite.
Kepler s third law equation derivation time period of satellite revolution.
Solar culmination and equation of time.
You can calculate the speed of a satellite around an object using the equation.
Sunrise and sunset times location sunrise and sunset times major cities.
The equation is independent of mass.
For objects in the solar system this is often referred to as the sidereal period determined by a 360 revolution of one celestial.
Artificial satellites and.
The orbital period is the time a given astronomical object takes to complete one orbit around another object and applies in astronomy usually to planets or asteroids orbiting the sun moons orbiting planets exoplanets orbiting other stars or binary stars.
Near the earth surface time period of the satellite.
T 2π r 3 gm 2π r h 3 g g gm r 2.
The period of the earth as it travels around the sun is one year.
We will derive the equation for kepler s third law using the concept of period of revolution and the equation of orbital velocity.
Calculates the orbital radius and period and flight velocity from the orbital altitude.
Geostationary or parking satellites.
This is the first equation or formula of orbital velocity of a satellite.
In this process the equation of time period of revolution of earth satellite would be derived as well.
Where t is the period of the satellite r is the average radius of orbit for the satellite distance from center of central planet and g is 6 673 x 10 11 n m 2 kg 2.
T 2π r 3 gm 3π gp.
The period of a satellite t and the mean distance from the central body r are related by the following equation.
Time period t circumference of the orbit orbital velocity.